The document discusses rotational motion and kinematics. It defines key concepts like the radian, angular velocity, and angular acceleration. It describes how to relate linear and rotational motion through equations. It also introduces the concept of moment of inertia, which describes an object’s resistance to changes in rotational motion based on its mass distribution. Different formulas are given for calculating the moment of inertia of objects like rods, disks, and point masses rotating around different axes.
3. The radian Half a radian would subtend an arc length equal to half the radius and 2 radians would subtend an arc length equal to two times the radius. A general Radian Angle ( ) subtends an arc length ( s) equal to R. The theta in this case represents ANGULAR DISPLACEMENT.
4. Angular Velocity Since velocity is defined as the rate of change of displacement. ANGULAR VELOCITY is defined as the rate of change of ANGULAR DISPLACEMENT .
5. Translation vs. Rotation Translational motion tells you THREE THINGS • magnitude of the motion and the units • Axis the motion occurs on • direction on the given axis Example: v =3i This tells us that the magnitude is 3 m/s, the axis is the "x" axis and the direction is in the "positive sense". Rotational motion tells you THREE THINGS : • magnitude of the motion and the units • the PLANE in which the object rotates in • the directional sense ( counterclockwise or clockwise) Counterclockwise rotations are defined as having a direction of POSITVE K motion on the "z" axis
6. Rotation Example: Unscrewing a screw or bolt = 5 rad/sec k Clockwise rotations are defined as having a direction of NEGATIVE K motion on the "z" axis Example: Tightening a screw or bolt = -5 rad/sec k
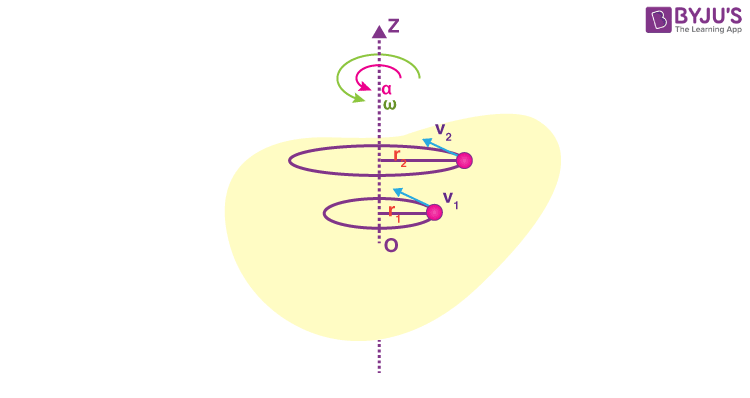
7. Angular Acceleration Once again, following the same lines of logic. Since acceleration is defined as the rate of change of velocity. We can say the ANGULAR ACCELERATION is defined as the rate of change of the angular velocity. Also, we can say that the ANGULAR ACCELERATION is the TIME DERIVATIVE OF THE ANGULAR VELOCITY . All the rules for integration apply as well.
8. Combining motions –Tangential velocity First we take our equation for the radian measure and divide BOTH sides by a change in time . The left side is simply the equation for LINEAR velocity. BUT in this case the velocity is TANGENT to the circle ( according to Newton’s first law ). Therefore we call it TANGENTIAL VELOCITY . Inspecting the right side we discover the formula for ANGULAR VELOCITY. Therefore, substituting the appropriate symbols we have a formula that relates Translational velocity to Rotational velocity.
9. Tangential acceleration and rotational kinematics Using the same kind of mathematical reasoning we can also define Linear tangential acceleration . Inspecting each equation we discover that there is a DIRECT relationship between the Translational quantities and the Rotational quantities. We can therefore RE-WRITE each translational kinematic equation and turn it into a rotational kinematic equation.
10.
11.
12. Moment of Inertia, I m1 m2 Consider 2 masses, m1 & m2, rigidly connected to a bar of negligible mass. The system rotates around its CM. This is what we would see if m1 = m2. Suppose m1>m2. m1 m2 r1 r2 Since it is a rigid body, the have the SAME angular velocity, The velocity of the center, v cm of mass is zero since it is rotating around it. We soon see that the TANGENTIAL SPEEDS are NOT EQUAL due to different radii.
13.
14. Example A very common problem is to find the velocity of a ball rolling down an inclined plane. It is important to realize that you cannot work out this problem the way you used to. In the past, everything was SLIDING. Now the object is rolling and thus has MORE energy than normal. So let’s assume the ball is like a thin spherical shell and was released from a position 5 m above the ground. Calculate the velocity at the bottom of the incline. 7.67 m/s If you HAD NOT included the rotational kinetic energy, you see the answer is very much different.
15. Example: Moment of Inertia, I m1 m2 Let’s use this equation to analyze the motion of a 4-m long bar with negligible mass and two equal masses(3-kg) on the end rotating around a specified axis. EXAMPLE #1 -The moment of Inertia when they are rotating around the center of their rod. EXAMPLE #2 -The moment if Inertia rotating at one end of the rod m1 m2 24 kgm 2 48 kgm 2